Large Deflections of Buckled Bars

This post is the first of a new category of application scope examples for Ple4Win. The emphasis of these examples is using the program ‘beyond the box’. See the starting page for more information.

Large Deflections of Buckled Bars

Consider a slender rod, as shown in figure 1. It is fixed at the base, free at the upper end.

Figure 1: Vertical bar, fixed at the base.

If a load P is applied that is somewhat larger than the critical value described by Euler’s Column Formula:

with

then a large deflection of the bar is produced. As described in Timoshenko & Gere’s “Theory of elastic stability” (chapter 2.7 “Large deflections of buckled bars (The Elastica)”) the relation between applied load and deflection can be described using the formula:

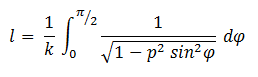
with

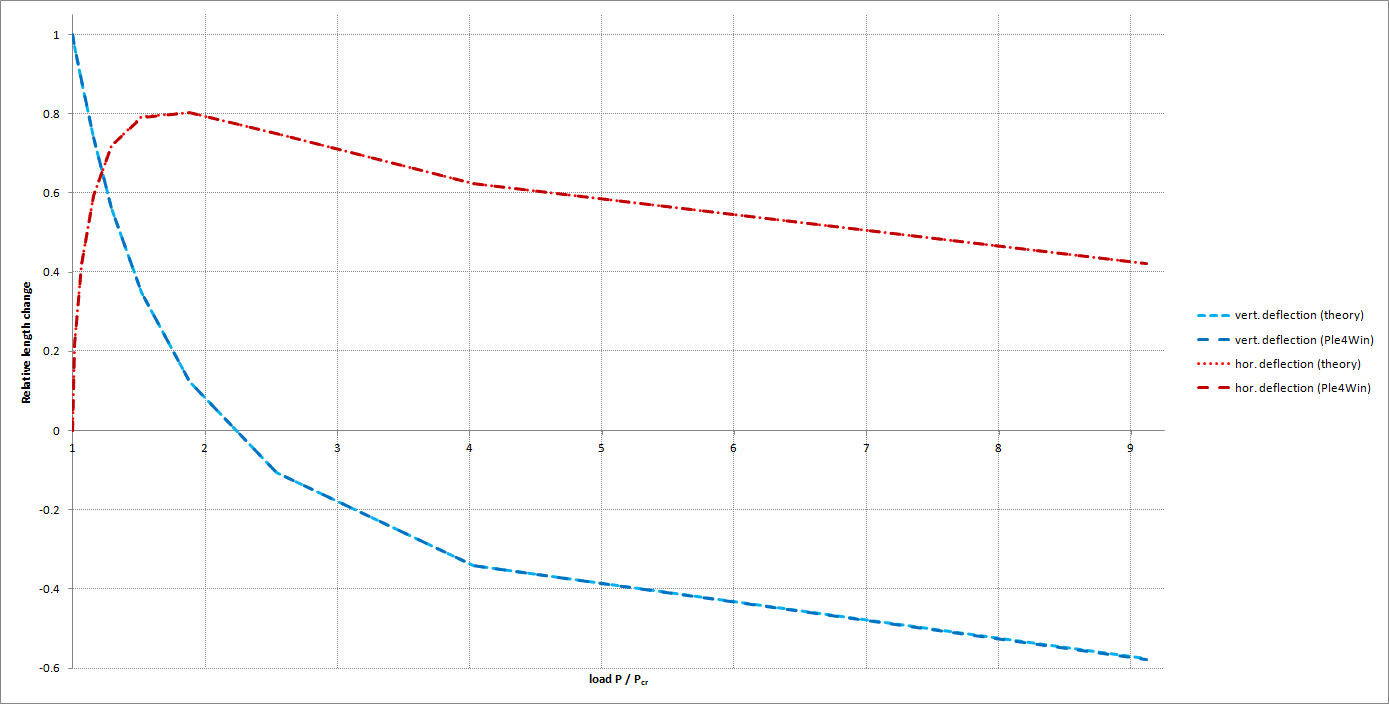
With Ple4Win, it is possible to calculate this beam deflection behaviour. Figure 2 shows the calculated bar deflections at different ratio’s P / P_cr

Figure 2: Deflections of a vertical bar at different ratio’s P / P_cr,

Note that the “non-linear geometry model” option must be used as well as the “linear material model” option. As the theory does not take compression effects or the influence of shear force into account, the material parameters in Ple4Win must be adapted to minimise their influence (these effects can not be switched off in Ple4Win).

Figure 3 shows a comparison between vertical and horizontal deflections as calculated by the program and as mentioned in the book by Timoshenko & Gere. The deviations are less than 2% for an angle  of 20° (compression effects) and between 0.1% and 0.5% for angles of 40° and higher.

Figure 3: Comparison between theoretical calculations and results obtained from Ple4Win.